Project leader: Professor Demetre Kazaras
Project manager: Kai Xu
Team members: Hiba Benjeddou, Ben Goldstein, Khiyali Pillalamarri, Nico Salazar
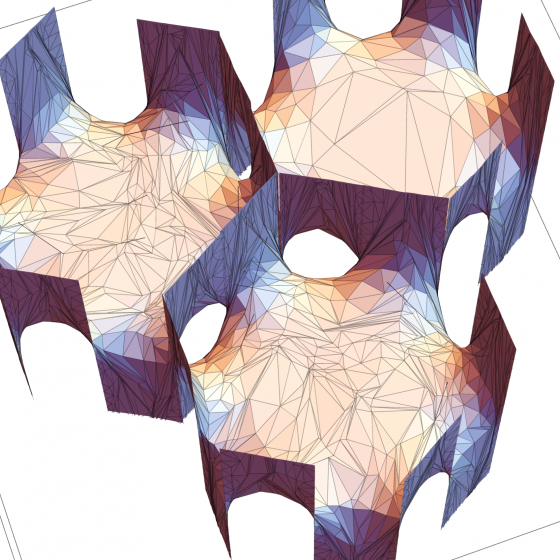
A minimal surface is a surface in 3-space which locally minimizes surface area. These structures are found in nature as membranes experiencing equal pressure from opposing sides, such as soap films spanning a wireframe. In our project we investigated and found new examples of 'minimal mod-3 surfaces'. These objects are made up of pieces of minimal surfaces meeting each other 3 at a time along 'singular curves' so that the angles between colliding faces are always 120 degrees. Not much is known about these minimal mod-3 surfaces -- our goal was to find new examples and investigate what properties the singular curves might have.
We first considered surfaces of revolution, obtained by rotating appropriate collections of catenary curves. Infinitely many examples were found, and their singular curves display a rich structure. Another class was constructed with a 'screw-motion symmetry' by carefully arranging helicoidal surfaces to intersect at 120 degrees. Our third approach was to design wire-frames with special symmetry so that the minimal-area surface spanning them could be extended to all of 3-space by rotation and translation. We also investigated the intrinsic geometry of the mod-3 surfaces themselves, understanding how distances behave on a particular example called the 'round mod-3 disc'.