Project leader: Professor Robert Bryant
Project manager: Yuhao Hu
Team members: Sandra Batakana, Zephyr Farah, Qinzhe Liu
Project summary:
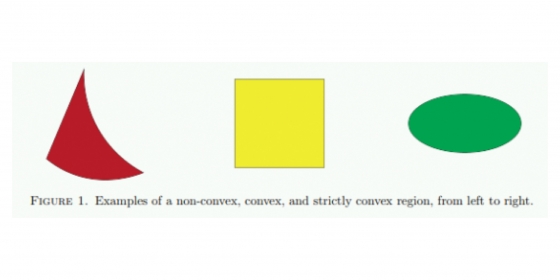
Our project uses geometric concepts to explore the properties of strictly convex curves and shapes of “constant width.” That is, regardless of how they are turned, they fit in-between two parallel lines or planes separated by the width w of the object. The most trivial examples are the circle and the sphere. However, there exist many other constant width curves in IR2 (and surfaces in IR3) such as Reuleaux polygons and the motor of the Wankel engine. Given that these curves and surfaces are strictly convex, we utilized a support function and its Fourier series to describe the movement of a supporting line S(θ) around the curve (or surface). We looked at how convex figures can be inscribed in convex polygons such as a triangle whose angles are rational multiples of a right angle, so that they touch all sides, no matter the orientation of C (a property called equi-inscribability). We find (in the IR2 case) the range(s) for the external angles of any n-gon (where n ≥ 3) and the corresponding symmetries of the curves of constant width that can be equi-inscribed in them.